
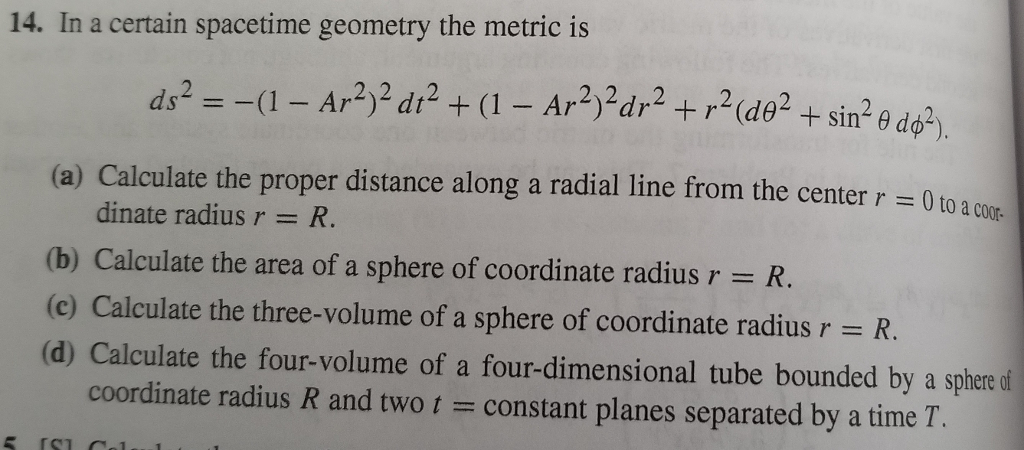
Exercise 10.1.1 Sketch the curve described by the parametric equations x(t) 3t + 2, y(t) t2 1, for 3 t 2. The starting point and ending points of the curve both have coordinates (4, 0). In the first case, the z coordinate is always 0, so this describes precisely the unit circle in the x - y plane. 1, 2, 3 + t 1, 2, 2 1 + t, 2 2 t, 3 + 2 t. In a similar fashion, we can graph a curve that is generated by a function r f(). This is the graph of a circle with radius 4 centered at the origin, with a counterclockwise orientation. As t varies, the first two coordinates in all three functions trace out the points on the unit circle, starting with ( 1, 0) when t 0 and proceeding counter-clockwise around the circle as t increases.

In GR, there is no underlying coordinate system in which spacetime curves for the same. In the rectangular coordinate system, we can graph a function y f(x) and create a curve in the Cartesian plane. We introduce the path or arc length coordinate, s, which measures the distance traveled by the particle along the curved path. The line segment starting from the center of the graph going to the right (called the positive x-axis in the Cartesian system) is the polar axis. Not that we know of, and not that it is important for general relativity. The curve in space described by the particle is called the path, or trajectory. In Minkowski spacetime we usually use inertial frame coordinates (t, x, y, z) since. Sometimes the equation of a surface in Cartesian coordinates can be transformed into a simpler equation in some other coordinate system, as in the following example.\): The polar coordinate system. Consider a particle moving in a circular orbit of radius r about a. Figure 1.7.5: Spherical coordinate surfacesįigures 1.7.4(a) and 1.7.5(a) show how these coordinate systems got their names.
Φ_0\), we see from Figure 1.7.5 that the surface \(ρ = ρ_0\) is a sphere of radius \(ρ_0\) centered at the origin, the surface \(θ = θ_0\) is a half-plane emanating from the \(z\)-axis, and the surface \(φ = φ_0\) is a circular cone whose vertex is at the origin.


 0 kommentar(er)
0 kommentar(er)
